Luck is a byword for a random process, which is something that happens without method. If you cannot find a method or pattern behind an event, you can consider it random and therefore impossible to predict.
The inverse of luck is skill – using knowledge or abilities to influence an outcome. Successful betting essentially comes down to the accurate assessment of the influence of skill vs luck within the context of an event, and where skill is present, accurately assessing (also known as handicapping) the relative attributes of the participants.
A coin-toss or the roll of a dice are both entirely random processes, which is why they are used as arbitrary methods to decide who kicks-off, serves or starts in a game of Monopoly. A coin or die have no memory, so one toss or roll doesn’t influence the next. They can be fun to bet on, but you can’t take a systematic approach, so when you bet on random events and win, you are lucky because no skill is involved.
Contents
How can you measure the influence of luck?
Though the outcome of a coin-toss is random, the distribution of outcomes over the long term isn’t, because we know the probability of the outcomes and random events confirm to what we call the bell curve (this is known as the law of large numbers).
You can measure how likely things are correlated by something called a P-Value, which gives the probability that random chance could explain the result, but that is quite abstract and hard to grasp. To understand the extent to which an event can be influenced by luck, you have to assign values to luck and skill and then see how they play out over increasing repetition; the Two Jar Model is a great entry-level way to visualise this.
What is the Two Jar Model?
Imagine you have two jars filled with balls. One jar represents Luck and the other Skill. Balls in the Skill jar have a minimum value of 0 equivalent to no skill, +1 and +2. The other jar, representing Luck, can have values ranging from -3 (bad luck), 0 (no luck) and +3 (good luck). The idea is to draw an equal number of balls from the jars and add the scores together – consider scores above 0 to be good outcomes.


Table 1 illustrates the possible outcomes for a single draw, where the range of good outcomes outweigh the bad, but even though high skill is present at that frequency extremely bad luck can still dominate. (For a more in-depth analysis of this, read Joseph Buchdahl’s excellent ‘Squares & Sharps, Suckers & Sharks’)
This analogy is simplistic but once you consider the impact of repeatedly drawing balls from each jar, it demonstrates that in non-random events where the skill factor is greater than luck, the influence of skill will increase over repetitions. This should start to make sense for bettors when they consider the common formats for sporting events and the prevalence of big upsets.
Applying the Two Jar Model to sports betting
Sporting events are structured to enable the more skilled performer to ‘rise to the top’. In everything from the World Snooker Championship to differing formats of tennis majors or UFC Championship fights, as rounds progress, or where the event is of a higher status, the result is determined after increasing repetitions as a means to enable skill to be the dominant factor.
The example of tennis majors
If we look at tennis as an example, it is evident that skill plays a greater role than luck, but that luck is present, whether in line calls, the influencer of weather, or where the ball falls when hitting the net-chord. There is an argument to say that luck plays a role if lesser skilled players use a higher % of risky passing shots, or faster second serves.
A game is just a series of points where – just like with the Jar example – relative skill is the dominant factor but luck is also present, the proportions of each are in relative to the perceived luck/skill bias (based on the odds). But the outcome would never be decided by just drawing one ball from each jar (the equivalent of a single-point match) because it maximises the chance that luck is the defining factor.
The influence of skill on the outcome of a tennis match will increase as the number of repetitions (points) increase, and a study by Chris Gray in a 2015 edition of the magazine Significance helps illustrate this.
Independence & modelling
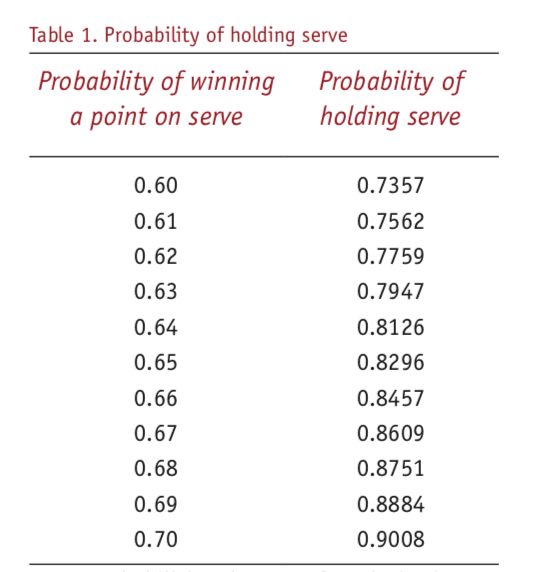
Based on data for the top 50 players (from 2014), Gray illustrates that the probability range of winning a point on serve was roughly between 60% and 70%. This translates into a probability of holding serve of between 73.6% and 90.0%, which is shown in Table 1.

If players are of equal skill, the way a point should pan out is pretty obvious (see Table 2).
For example, players that both have the probability of winning points on serve of 0.6, have the same probability of winning a set: 0.5 or 50%.
What is immediately obvious (and relevant) is that the probability of winning a set is greater than the point differentials. This should be intuitive: the longer the match, the more time there is for the better player to prevail, which is what we learned in the Jar exercise.
However, because our players are of equal skill our understanding of the influence of luck comes into play as it can become the difference between the two players.
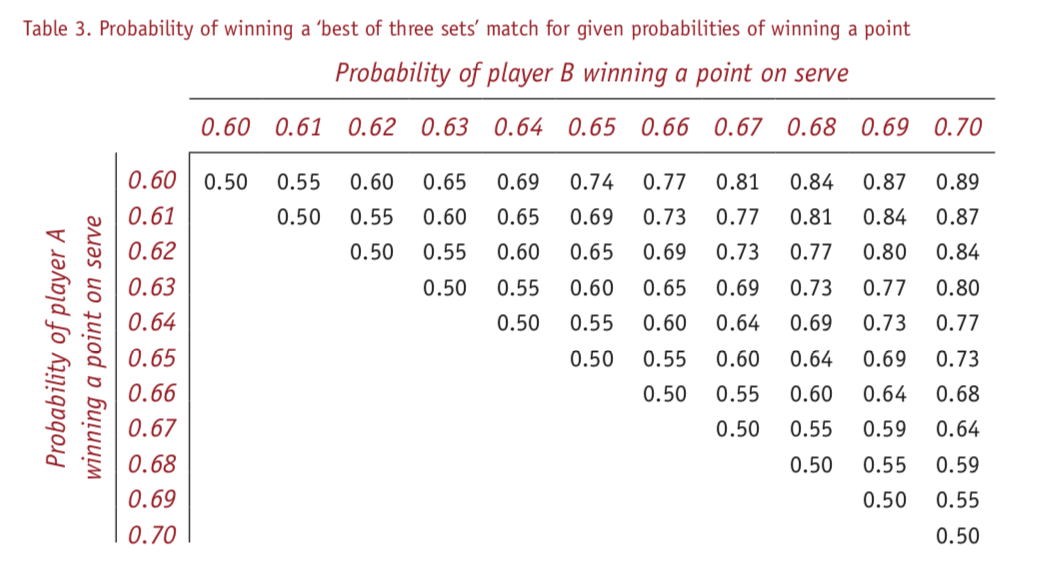
Looking at the most extreme skill differential where player A has 0.70 chance of winning on serve versus player B with only 0.60 chance the better player only wins an individual set 79% of the time, but as the scale extends to a best-of-three match and the better player will win 89% of the time.
As we already mentioned the most important events increase repetitions, and so it is with tennis Grand Slams where the format (for men) moves from best-of-three to best-of-five. According to Gray:
Consider players with probabilities of winning points on serve of 69% and 61%. This translates into the favourite having a 74% chance of winning a set and 90% of winning the match. This would be common of a top 10 player against a player ranked between 70 and 100. Using the model and the parameters, if they are two sets behind, they still have a 42% chance of making the big comeback.
Key takeaways
What Gray shows, with real world data, is exactly the same as the simple jar analogy. Skill has an increasing influence over time/repetition. So what are the take-outs for bettors:
Favourites win more often in Grand Slams than standard ATP events because the longer format accentuates the skill differential. Conversely, more shocks will occur in standard ATP events (Bo3), while for the WTA the prevalence should roughly be the same (because they are always Bo3).
Once you grasp the concept you can extend the logic to all areas of sport and explain changing patterns in format and outcome. Think about the preference for short format events like cricket (20/20) and snooker (6-Red), which produce more compact, exciting and unpredictable results. Snooker especially has come a long way, in 1952 the final was the best of 145 frames!
The concept of luck and repetition may also explain why Wimbledon has produced two epic encounters in recent years. Because of the increasing suitability of the surface to fast servers, the length of points has been reduced – essentially reducing repetition within a point and therefore the role that luck might play per point – turning a match-up of very evenly matched big servers down into a simple war of attrition. This has now resulted in a change of format.
You can also start to search for events where luck can – in the right circumstances – have a greater influence, or where luck can change the parameters of the game. Golf is a great example. When players tee-off is entirely random, but can have a dramatic influence because of the differing weather conditions players encounter when looked at in the single event context (this will be significant within an event but would be expected to average out over a season). This is especially true at venues where weather is volatile such as the British Open, played on a links course.
The field of play is also an order of magnitude greater for golf compared to other sports, providing opportunities for luck, and interaction with a complex environment.
Exceptions & anomalies
Penalty kicks are a very strange twist on the skill/luck dynamic. To decide a stalemate in 90 minutes, regular game time is extended by 30%, essentially extending the chance of the better team winning, but if that isn’t enough to allow a result, the format switches asymmetrically to a format where luck suddenly has a far greater influence. On top of this you have to consider the context of a one-off game, where with so much as stake loss-aversion can influence tactics and therefore skew ideas of how skill should prevail over luck.
Hopefully this article – and the simple Two Jar analogy – has enabled you to think differently about the role of luck in sport and how you might account for it in betting. Where skill influences the outcome of an event more than luck, that influence will be greater over time.
TL’DR – Proverbs often provide an eloquent shorthand way of boiling down complex subjects, and in this case ‘Cream rises to the top’ applies.
Now that you know all there is to know about the role of luck in betting, head over to our sportsbook and test out what you’ve learnt today!





