Luck refers to something that is random, that is, something that happens without rules. If there is no method or pattern behind an event, it is random. In other words, it is impossible to predict. So, what is the opposite of luck? It is skill. That is, something that you can actually influence the outcome with your knowledge and ability. To conquer betting, you basically need to consider how much skill and luck you have, and if you determine that skill is effective, then properly evaluate (also known as handicap ) the attributes of the players and events before deciding to bet. This is a sure-fire way to win. Coin tosses and dice rolls are completely random. That is why they are used in all sports as the best way to decide who will kick off, who will have the right to serve, etc. Coins and dice have no memory, so one toss or dice roll has no effect on the next throw. It’s fun to bet on things like this, but you can’t take a strategic approach, so when you win by betting on a random event in a single shot, this is what we call “lucky” because there is no skill involved.
Contents
How to measure your luck
The outcome of a single coin toss is completely random, but the long-term probability of doing it over and over again is not. The reason is that the probability of an outcome can be calculated, and successive random events follow a law called the bell curve (also known as the law of large numbers). We can measure how correlated things are with a value called the “P value”, which indicates the probability of each possible outcome of a random event. However, this is quite abstract and difficult to understand. To understand how much luck influences an event, we need to assign values to luck and skill and see how they behave as the sample grows.
The “two bottles” theory
Imagine there are two jars filled with balls. The first jar is “luck” and the other is “skill”. The balls that go into the skill jar are 0 (zero skill), plus 1 or plus 2. The other “luck” jar has three balls: minus 3 (very bad luck), 0 (unlucky), and plus 3 (lucky). Then, draw the same number of balls from each jar and add up the scores. A score of 0 or more means a positive result.


This table shows the possible outcomes for a single jar. Here, the range of positive outcomes outweighs the negative outcomes, but even if high skill is present at that frequency, extreme bad luck can still make it negative. (For a deeper look , I recommend reading Squares & Sharps, Suckers & Sharks by Joseph Buchdahl.) This analogy is simple, but it shows that as balls are repeatedly drawn from each jar, the element of skill outweighs luck, i.e., in a non-random event, the effect of skill becomes increasingly greater with each iteration. This is best understood by considering the typical format of a sports competition (number of games, number of sets, etc.) and how many upsets are possible.
Applying the “two jar” theory to sports betting:
Sports competitions are fundamentally structured to allow the more skilled performers to “come out on top.” From the snooker world championships to tennis majors to UFC championship matches, the outcome is determined by skill rather than luck as the rounds progress or by repeated play in high-status competitions.
Tennis Example
For example, in tennis, skill is clearly a bigger factor than luck, but there is still some luck involved, such as the line judgment, the weather, and where the ball lands on the net. Luck can also play a role when a less skilled player tries to take more risky passing shots or a faster second serve. As with the jar example, in sports where skill is a big factor but luck is also a factor, the percentage of each depends on whether luck or skill is prioritized (based on the odds). Points in a sports match are just repetitions. However, the result is not decided by simply drawing balls one by one from the jar (corresponding to a one-point match). If the result was decided in one repetition, then luck would be the deciding factor. The influence of skill on the outcome of a tennis match increases with the number of repetitions (points). A study by Chris Gray in a 2015 article in the magazine Significance explains this well:
Gray’s Study
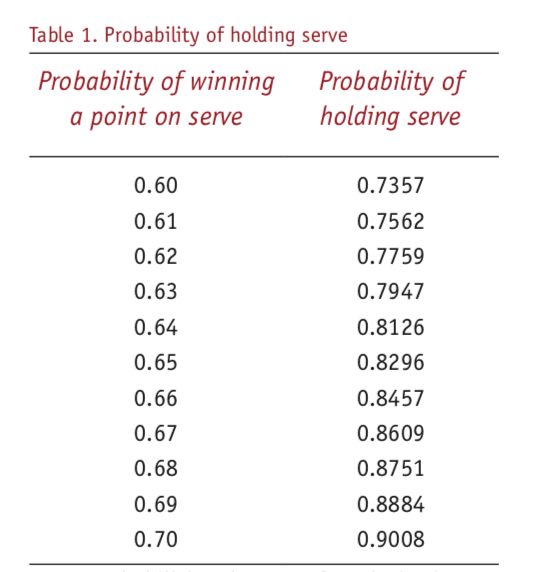
Based on data from the top 50 players (2014), Gray has produced statistics showing that the probability of winning a point on serve (Table 1, left) is approximately 60% to 70%, and the probability of holding a service game (Table 1, right) is 73.6% to 90.0%.

Table 2 shows the service game winning percentage based on the probability of winning a point on serve for Player B (horizontal axis) and Player A (vertical axis). If both players have the same level of skill, it is clear how the outcome will play out. For example, if both players have a 0.6 probability of winning a point on serve, then the probability of winning the service game is also the same, 0.5 or 50%. What we can see here is that the probability of winning a service game is greater than the difference in point winning percentage. This is intuitively clear from the bottle theory mentioned earlier, but the longer the match, the more samples are taken and the higher the probability that the better player will win. However, since the players’ skills are equivalent, it is important to understand that luck also plays a role, and it can be said that luck is the difference between the two players.
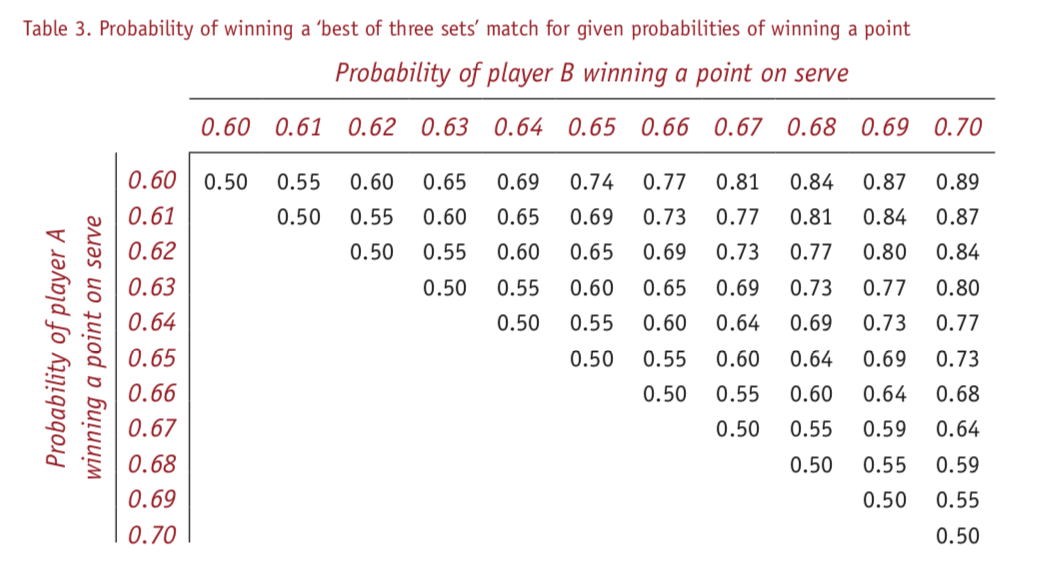
As an extreme example, if player A has a 0.70 probability of winning a serve and player B has a 0.60 probability of winning, then player A has a 79% chance of winning a set, but in a best-of-three match, that number rises to 89%. As we have already said, the more important the tournament, the more repetitive it becomes. This is also the case in tennis Grand Slams, which have moved from a best-of-three to a best-of-five format. According to Gray: “Consider two players who win points on serve 69% and 61% of the time. This means that a 69% player will win a set 74% of the time and win the match 90% of the time. It is common for top-10 players to play against players ranked 70-100. Using this model and parameters, if you are down two sets, you have a 42% chance of a comeback.”
summary
Gray uses real-world data to show exactly what the bottle analogy is about. Skills become more effective over time and repetition. Here are some key takeaways: Favourites win more often in Grand Slams than in standard ATP tournaments, because the longer match formats make the difference between players more pronounced. Conversely, standard ATP and WTA tournaments (best of three sets matches) allow for more surprises. Once you understand the theory, you can apply it to any sport and derive patterns of outcomes depending on the format. Think of shorter formats like cricket (20/20) or snooker (6red), where unpredictable outcomes can occur in more compact competitions. Snooker in particular can be a long journey, like the 1952 final, which was 145 frames long. This concept of luck and repetition, combined with two recent epic Wimbledon matches, may make sense. The court surface is becoming more suitable for fast servers, which reduces the length of points and therefore the repetition within a point, thus turning the role of luck per point, i.e., between very evenly matched big servers, into a simple war of attrition. This has led to changes in match format (best of three matches to best of five). It can also be used to look for events where luck has a greater impact or where it changes the parameters of the game. Golf is a very good example. The timing of when players tee off is completely random, but for an event it can be dramatically affected by the weather on that day (this is important on an event basis, but is expected to average out over the season). This is especially true in venues with variable weather, such as the British Open, which is played on links. Golf courses are also orders of magnitude larger than other sports, and are environments where complex factors interact with luck.
Are there any exceptions?
For example, penalty kicks are very difficult and can upset the balance between skill and luck. In order to get a good 90-minute decision, the normal match time is extended by 30%, which basically increases the chances of the favored team winning, but if the match is still not decided, suddenly the format switches to one where luck plays a much bigger role. On top of that, you have to take into account that it is a one-off match, and the desire to avoid losing your bet can affect tactics, which can distort the idea that skill should prevail over luck. I hope this article and the simple “two jars” analogy have given you a better understanding of the role of luck in sports and how to think about it in betting. The point is, if the outcome is influenced by skill rather than luck, remember that the influence will increase over time. Summary – A very simple way to summarise this complex topic is to use the proverb “Cream rises to the top” – this means that the best will come out sooner or later. Now that you know all about the role of luck in betting, go to a sportsbook and put what you have learned today into practice!